
\[V = -\frac\) may be written instead merely as \(|lmn \rangle\). The time-dependent Schrdinger equation is a partial differential equation that describes how the wavefunction evolves over time, while the time-independent. Abstract: Electronics industry is a major user of Metal. The expression for the potential energy of a hydrogen-like atom to be substituted for \(V\) in Schrödinger's equation is This equation is called the Schrödinger time-dependent equation. Wavelet solution of the time independent Schrdinger equation for a rectangular potential barrier. The expression for \(\nabla^2\) is spherical coordinates is lengthy and can be found mathematical and many physics or chemistry texts. It begins with the assumption that the wavefunction can be written as a product of two functions: one depending on the coordinate and one on time. Transcribed image text: Solve the time-independent Schrodinger equation for a particle of mass m and energy E > V0 incident from the left on the step.
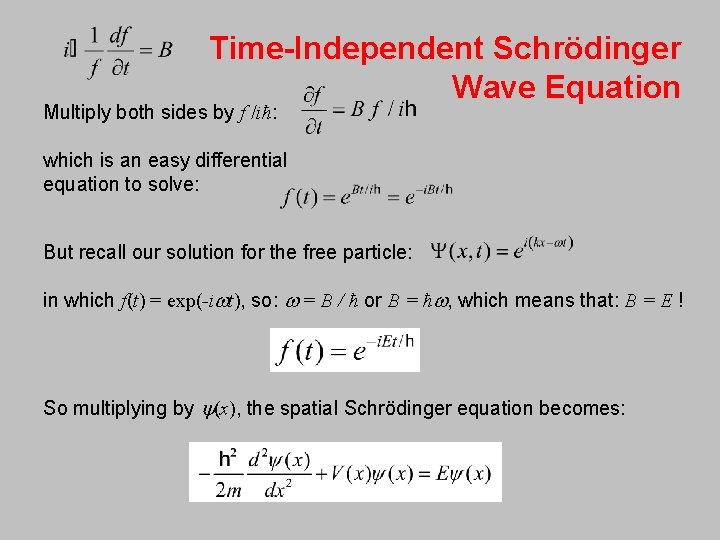
That is not 100% correct, as you see.The Schrödinger equation is best written and solved for atoms in spherical coordinates. The time-independent 1-dimensional Schrdinger equation is the governing equa- tion for determining the wavefunction, u(x, t) exp(iEt/h) of a single non-. The derivation of the time-independent Schrdinger equation doesn't assume both sides equal a constant. This motivates the choice of name for E: Physically, the eigenvalue of the Hamiltonian is the energy. H ( r) E ( r), which as you observe can be viewed as an eigenvalue equation for H. Thus if a general state is to be time dependent, as the Schrödinger equation implies, then the time dependence must reside in the expansion. For the spatial part, we find the time-independent Schrödinger equation. 2, so there is no reason to expect the eigenvectors themselves to carry any time dependence.
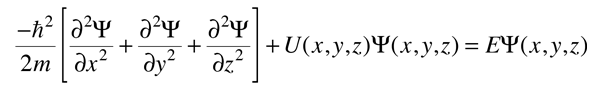
¸ w¹ is the Laplacian Operator in spherical coordinates.Every book says that the phase of the wave function has no physical meaning. The eigenvectors of a time-independent Hamiltonian come from the diagonalization procedure we used in Chap. is the solution to the Time Independent Schrodinger Equation in spherical coordinates: 2 2) 2 E m r Where, 2 22 2 1 sin sin r r T I w This operator gives the total energy of the quantum system. $$\frac$ play the most important role, since they give rise to interference patterns.Ĭomment: Feynman is quoted to have said "One of the miseries of life is that everybody names things a little bit wrong, and so it makes everything a little harder to understand in the world than it would be if it were named differently." It is quite similar here. chapter 3 The Time-Independent Schrdinger Equation For a particle of mass m in a potential V, the time-independent Schrdinger equation is written as where. The Schrödinger equation can be expressed as time-independent form as shown below, H E.

TIME INDEPENDENT SCHRÖDINGER EQUATION FREE
In my notes, I have the Time Independent Schrodinger equation for a free particle Table 6: Runtime and memory required to solve the Schrdinger equation with both a time- independent and a time-dependent Morse potential, using an 8th order. The time-independent Schrödinger equation predicts that wave functions can form standing waves, called stationary states (also called 'orbitals', as in atomic orbitals or molecular orbitals).These states are important in their own right, and if the stationary states are classified and understood, then it becomes easier to solve the time-dependent Schrödinger equation for any state.


 0 kommentar(er)
0 kommentar(er)
